Introduction

This is our fourteenth Article and the second of five of a ‘mini-series’ on bond markets. In China, individual investors tend to prefer to invest in equities and real estate. But the bond market is a sector that we expect to grow in the coming years along with bond funds so that more individuals will invest in this sector. This second article of five, is intended to explain and illustrate the valuation methodologies for fixed income investments. The following Article 15 on the yield curve is very important to this topic:

15. Understanding the Yield Curve

16. Credit Risk and Corporate Bonds

17. Structured Securities

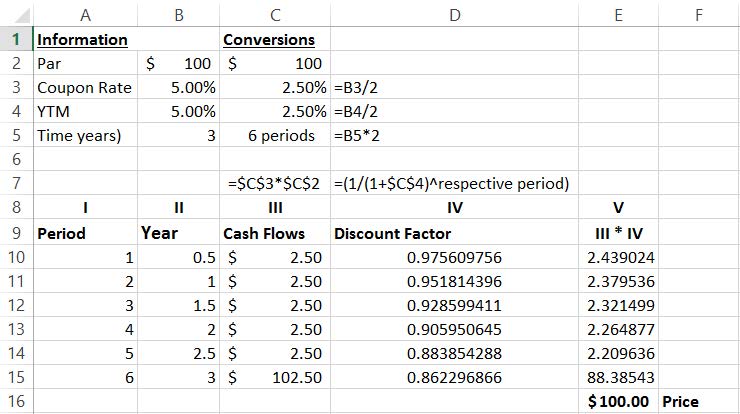
Present Value Calculations

In Article 11, we showed how to value a company using ‘discounted cash flow’ (DCF) method. The concepts of Present Value, Future Value, rates of return, are essential to finance. Bonds and money market instruments apply this concept of present value extensively. Just to review:

The price (value or present value) of a security is determined by forecasting what future cash flows will be earned, on what date and the appropriate rate of return/interest to discount them back to their current (present) value. Every bond and money market instrument is valued using that exact method. When teaching this in an academic program, tutors often simplify things to ‘whole years’ or ‘half years’, but we cannot do this in the real world.

Bond and money markets are based on ‘conventions’ or rules about how to calculate values of accrued interest. There will be ‘day-count conventions’ that stipulate the actual way you will calculate interest and, thus, price and your investment return. In every market I know about, we have a daily settlement convention. Thus, if you but or sell at 10.53 on a Tuesday, you will calculate the accrued interest in exactly the same way as if you had sold it at 14.12 on that same day. China uses this same-day settlement convention as well.

In illustrating these markets, we will look at three examples:

1. Money market – interest bearing instrument
2. Money market – discount security
3. Bond market – government bullet bond (as described in Article 13)

We will look at corporate bonds and credit risk in Article 16.

Money Market Securities

The money markets include debt instruments with an original maturity of 12 months or less. Interest in the money markets is typically paid in one single “bullet” at maturity. This makes the interest payable a simple-interest (as opposed to compound interest) calculation. The rate on the loan is normally quoted in percentage per annum, so the interest amount payable is pro-rated by the term of the instrument.

Note:

• PV is ‘present value’ or the current price
• FV is ‘future value’ are the legally contracted ‘bullet’ payment
• r is the rate of interest the market deems appropriate for this instrument and term
• #days is the number of days the investment is being held
• day count is what is specified by convention

This general formula can be used for either of the two money market instruments described. It is normal for banks to issue ‘certificates of deposit’ (CD) where a depositor (lender) gives the bank Par (100) and on the maturity of the receives bank the principal (Par) plus some additional interest. If it was a 4.0% CD for exactly one year, you would deposit 100 and get back 104 on maturity.

CDs are generally issued in very large amounts and so are not common to individual investors, they are more common with institutional investors. However, if you have invested in a money market fund, chances are that fund will have invested in bank CDs. We illustrate below with two of these instruments issued by the People’s Bank of China.

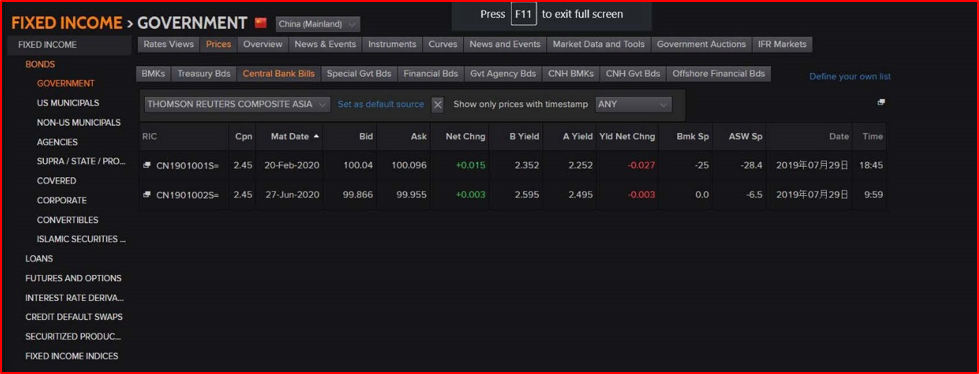
Using the top security to illustrate, here we see there is an interest-bearing CD that matures on 20 Feb 2020. The initial deposit would have been 100.00 (‘Par’) but on maturity you get repaid your principal (Par) plus a coupon payment of 2.45, or 102.45 in total. There is a second one with a longer maturity of 27 June 2020. Both, when they were first issued, would have had an initial term-to-maturity of one year or less.

We now look at another instrument, issued by the China government that is also short-term in nature but has a slight variation in terms to the bank CD. Refer below to the China government ‘T-bill’.

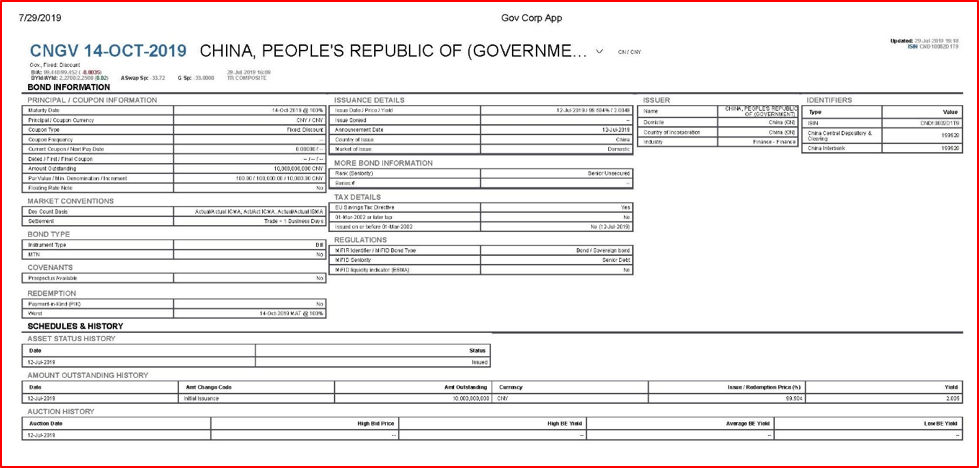
We can see the maturity date is 14-Oct-2019 and it matures at a value of 100 (Par) – there is no additional interest payment. But, if we look beside it, we see it was issued on 12-Jul-2019 at a price of 99.504. Thus, your ‘interest’ is that you are repaid 100 but paid less than that to buy it.

In summary, CDs are purchased (initially) at 100 (Par) and mature and pay a bullet payment that is greater than 100. Bills are bought at a discount to par and mature at par. Note, however, that an interest rate of, say, 5.0% is not the same investment return as a discount of 5.0%.

• 5.0 earned on par (100) is an investment return of 5.0% (5/100)
• 5.0 earned on 95 (discount price) is an investment return of 5.26% (5/95)

For historical reasons, financial institutions have offered CDs that are interest bearing and governments have issued (discount) bills. So, both types of instruments are common in most capital markets.

Bond Valuation

Since bonds have an initial term-to-maturity of greater than one year, they are most frequently ‘coupon bearing; there is one or more interim payments prior to the bond maturing. Again, we can use our simple Present Value method but in this case we have to PV a whole series of payments (not just one final payment) and then sum these together to get the value of the ‘bundle’ of cash flows. Though more complicated than money market, the fact that the interim payments (‘coupons’) are made in a consistent manner (annually or semi-annually) means that we can make these values quite quickly with either a calculator or an Excel spreadsheet. We use the example of the CGBs we referred to in Article 13, bonds issued by the China central government.

In the CGB market, bonds with an initial term-to-maturity of less than ten years pay an annual coupon. Bonds with an initial term-to-maturity of ten years or longer pay a semi-annual coupon.

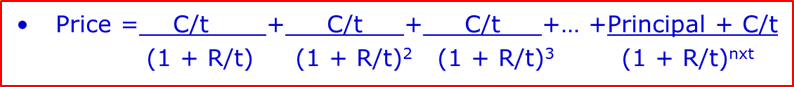
If we look at the five-year benchmark, we see the coupon is 3.19%. This is an annual coupon so each year we discount a cashflow of 3.19 and in the final year a cash flow of 103.19. But, if we look at the ten-year benchmark, we see a coupon of 3.29 but this is paid semi-annually. So, here we would need to discount 19 cash flows of 1.645 (3.29/2) and a final payment of 101.645.

It is important at this point to distinguish between ‘coupon’ and the ‘yield’ of a bond. When a bond is first issued, the coupon should reflect that correct yield to earn on that bond, for that category of issuer and for that maturity. But, after that point-in-time, things may change. The general level of interest rates in the market may go up or go down. We will talk about the ‘yield curve’ in more detail in Article 15. If this was a corporate issuer, its credit rating might go up or down meaning the correct yield for this bond may change, even if the general level of interest rates do not change. We will talk about credit risk and changes in Article 16. What we need to understand now, in general, is how the value of a bond changes when the ‘correct yield’ for that bond changes – the coupon is never changed after issuance.

Two important points follow from the pricing formula developed here:

1. The higher the required yield on the bond – i.e. the higher the rate used to discount its future cashflows – the lower will be its market price. This illustrates: Bond Market Law No 1: bond price varies inversely with yield.
2. The simple convention (‘yield-to-maturity’ or YTM) in the bond market is to apply the same rate to discount all of the bond’s future cashflows. (We will relax this assumption in Article 15 and talk about ‘realised compound yield’ or RCY, using different rates from the yield curve).

A starting point in bond investing is, thus, for your desired maturity, among similar credit rated issuers, among bonds of similar liquidity, which will provide you with the highest YTM. Just for illustration, I have confirmed the bond yield on the 3-year CGB in the below detailed analysis.

We can see this bond was first issued on 6 March 2019, when the 2.69% annual coupon was set. Now we see it is trading below its issue price at a bid price 99.484, which gives it a YTM of 2.8950%. I don’t believe there was any change in the credit rating of the government, so it appears that interest rates have risen since this bond was issued. Managing interest rate risk is one of the key tasks of investors in bonds.

Clean (Flat) vs. Dirty (Invoice) prices

A Clean or Flat price is the price of a bond without including the accrued interest that is due to the seller. Dirty or Invoice price is the legal amount you must pay the seller, which is both the price and accrued interest. Accrued interest is important to understand. As the owner of a bond, you are legally entitled to the accrued interest for all the days you owned the bond. Of course, interest is only paid in periodic coupons (annually for our 3-year example). If you bought this bond at issuance on 6 March 2019 and sold it to me on 29 July 2019, you would be entitled to 145 days of accrued interest and this amount (equal in price to 1.0584) would have to be added to the quoted price of 99.484 (or 100.5424) as proceeds to you. I pay you the accrued interest as you won’t be the holder when the next coupon is paid. The buyer always pays the seller of the bond any accrued interest. But – note- that quoted prices never include accrued interest (99.484), they are the Clean (Flat) prices only. So, why the inconsistency?

Imagine that we hold this bond through the next coupon date, while market conditions remain the same. As the coupon date approaches all the future cash flows get closer, so the present value of the bond rises steadily. Similar price drops would occur every time a coupon is paid.

To eliminate such ‘technical’ price fluctuations the markets quote bond prices on a clean (or flat) basis: they subtract from the bond’s present value the interest amount accrued since the start of the current coupon period (in the figure, the vertical distance between the bond’s present value and the horizontal timeline). Thanks to this technique, other things being equal, there should be no perceptible change in the bond’s quoted price as it goes through a coupon date. The clean price gives investors a measure of market value that is not affected by the payment of a coupon.

In Conclusion

This initial valuation module on bonds shows how different the investment analysis is for high-grade bonds as compared to equity markets. For equity investing there is a lot of fundamental analysis of economics, industry, company strategy and many other variables. For government (high grade) bonds it is a much more quantitative and technical form of analysis.

We will see in Article 16 that when a bond is issued by a very low rated entity, it sometimes takes on ‘equity-like characteristics’. But, always keep in mind the two fundamental differences between a bond and an equity investment as summarized in Article 13.

John D. Evans, CFA (author) has over 24 years’ experience in the international capital markets working with issuers of securities and investors around the world. He has designed and taught Master’s programmes in investment management at universities in the UK and China. He was most recently Professor of Investment Management at XJTLU in Suzhou. He now manages SEIML, a consultancy to early-stage companies in China.

Jina Zhu (translator) did her Master’s in Economics in France and is fluent in Mandarin, English and French. She also works at SEIML supporting early-stage companies grow and raise capital in China.

29 July 2019